Page 22 - Mathematics

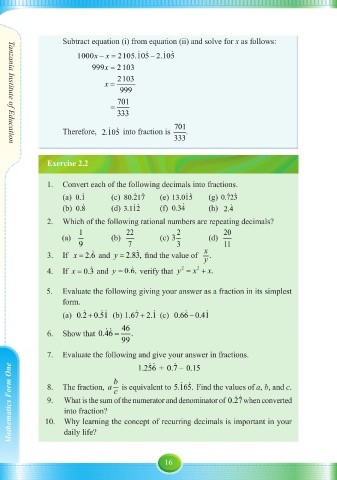
Subtract equation (i) from equation (ii) and solve for x as follows:

1000xx−= 2105.105 2.105−   
999x = 2 103

2103
x =
999
701
=
333
701
 
Therefore, 2.105 into fraction is 333 .

Tanzania Institute of Education

Exercise 2.2

1. Convert each of the following decimals into fractions.

 

 

(a) 0.1 (c) 80.217 (e) 13.015 (g) 0.723




(b) 0.8 (d) 3.112 (f) 0.34 (h) 2.4
2. Which of the following rational numbers are repeating decimals?
1 22 2 20
(a) (b) (c) 3 (d)
9 7 3 11
x


3. If x = 2.6 and y = 2.83, find the value of .
y


2
2
4. If x = 0.3 and y = 0.6, verify that y = x + . x
5. Evaluate the following giving your answer as a fraction in its simplest
form.



(a) 0.2 0.51+   (b) 1.67 2.1+  (c) 0.66 0.41− 

6. Show that 0.46 = 46 .
99
7. Evaluate the following and give your answer in fractions.

 
Mathematics Form One 9. What is the sum of the numerator and denominator of 0.27when converted
1.256 + 0.7 – 0.15
b
 
8. The fraction, a is equivalent to 5.165. Find the values of a, b, and c.
c
 
into fraction?
10. Why learning the concept of recurring decimals is important in your
daily life?

16

25/10/2024 09:51:05
Mathematics form 1.indd 16
Mathematics form 1.indd 16 25/10/2024 09:51:05