Page 153 - Physics

Pressure

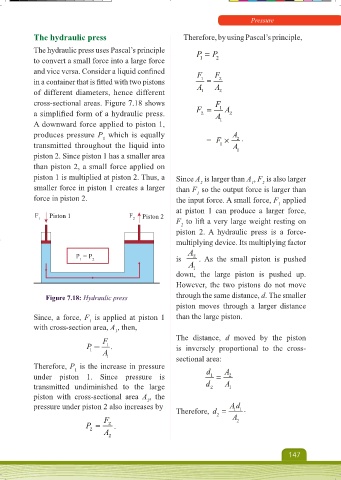
The hydraulic press Therefore, by using Pascal’s principle,
The hydraulic press uses Pascal’s principle P = P
to convert a small force into a large force 1 2
and vice versa. Consider a liquid confi ned F F
in a container that is fi tted with two pistons A 1 = A 2
of different diameters, hence different 1 2
cross-sectional areas. Figure 7.18 shows F 1
a simplifi ed form of a hydraulic press. F = A A 2
2
A downward force applied to piston 1, 1
produces pressure P which is equally = F × A 2 ⋅
1
transmitted throughout the liquid into 1 A 1
piston 2. Since piston 1 has a smaller area
than piston 2, a small force applied on
piston 1 is multiplied at piston 2. Thus, a Since A is larger than A , F is also larger
2
1
2
smaller force in piston 1 creates a larger than F so the output force is larger than
1
force in piston 2. the input force. A small force, F applied
1
at piston 1 can produce a larger force,
F 1 Piston 1 F 2 Piston 2 F to lift a very large weight resting on
2
piston 2. A hydraulic press is a force-
multiplying device. Its multiplying factor
A
P = P 2
1 2 is . As the small piston is pushed
A
1
down, the large piston is pushed up.
However, the two pistons do not move
Figure 7.18: Hydraulic press through the same distance, d. The smaller
piston moves through a larger distance
Since, a force, F is applied at piston 1 than the large piston.
1
with cross-section area, A , then,
1
F The distance, d moved by the piston
P = 1 . is inversely proportional to the cross-
1
A
1 sectional area:
Therefore, P is the increase in pressure d A
1
under piston 1. Since pressure is 1 = 2
transmitted undiminished to the large d 2 A 1
piston with cross-sectional area A , the
2
pressure under piston 2 also increases by A d
Therefore, d = 1 1 ⋅
F 2 A 2
P = 2 .
2 A
2

147

Physics Form 1 Final.indd 147 16/10/2024 20:57