Page 119 - Mathematics

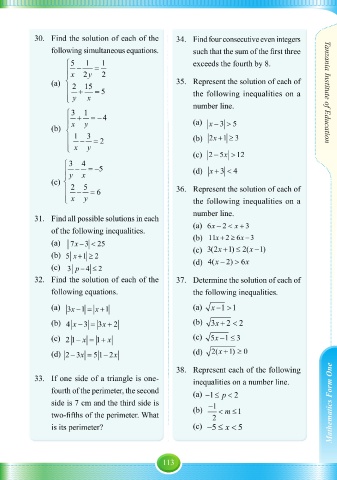
30. Find the solution of each of the 34. Find four consecutive even integers
following simultaneous equations. such that the sum of the first three

 5 1 1 exceeds the fourth by 8.
 x − 2y = 2

(a)  2 15 35. Represent the solution of each of
 y + x = 5 the following inequalities on a Tanzania Institute of Education


 3 1 number line.
 x + y = − 4 (a) x − 3 > 5
(b) 

1
3
 1 − 3 = 2 (b) 2x + ≥
 x y

(c) 2 5x− > 12
 3 4 5
 y − x = − (d) x + 3 < 4

(c) 
 2 − 5 = 6 36. Represent the solution of each of
 x y the following inequalities on a


31. Find all possible solutions in each number line.
2
x
of the following inequalities. (a) 6x −< + 3 3
3
(a) 7x−< 25 (b) 11x + 2 6x≥ − − 1)
1) 2(x≤
(c) 3(2x +
1
(b) 5 x+ ≥ 2 (d) 4(x − 2) > 6x
(c) 3 p − 4 ≤ 2
32. Find the solution of each of the 37. Determine the solution of each of
following equations. the following inequalities.

(a) 3x − = x + 1 (a) x −>
11
1
2
2
(b) 4 x − 3 = 3x + 2 (b) 3x +<
(c) 21 x− = 1 x+ (c) 5x − 13≤
(d) 2 3x− = 5 1 2x− (d) 2(x + 1) ≥ 0

38. Represent each of the following
33. If one side of a triangle is one- inequalities on a number line.
fourth of the perimeter, the second (a) 1 p−≤ < 2
side is 7 cm and the third side is − 1 Mathematics Form One

two-fifths of the perimeter. What (b) 2 < m ≤ 1
x
is its perimeter? (c) 5− ≤ < 5

113

25/10/2024 09:51:42
Mathematics form 1.indd 113
Mathematics form 1.indd 113 25/10/2024 09:51:42