Page 110 - Physics

Physics for Secondary Schools

Acti vity 5.4 (b) What is the weight of the stone when

Aim: To determine the relative immersed in water?
density of a stone using (c) Calculate the apparent loss in weight.
Archimedes’ principle. (d) Calculate the relative density of the
Materials: Stone, spring balance, water, stone.
beaker and string
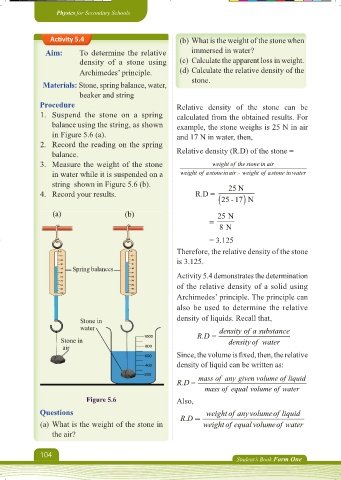
Procedure Relative density of the stone can be
1. Suspend the stone on a spring calculated from the obtained results. For
balance using the string, as shown example, the stone weighs is 25 N in air
in Figure 5.6 (a). and 17 N in water, then,
2. Record the reading on the spring
balance. Relative density (R.D) of the stone =
3. Measure the weight of the stone weight of the stone in air
in water while it is suspended on a weight of astoneinair - weight of astone inwater
string shown in Figure 5.6 (b). 25 N
4. Record your results. R.D = ( 25 - 17) N

(b)
( (a)a) (b) 25 N
=
8 N
= 3.125
Therefore, the relative density of the stone
is 3.125.
Spring balances
Activity 5.4 demonstrates the determination
of the relative density of a solid using
Archimedes’ principle. The principle can
also be used to determine the relative

Stone in
Stone in density of liquids. Recall that,
water density of a substance
water
R.D =
Stone in
Stone in densityof water
air
air
Since, the volume is fi xed, then, the relative
density of liquid can be written as:
density of a substanceme of liquid
mass of any given volu
RD
R.D = =
densityof waterume of water
mass of equal vol
Figure 5.6 Also,
Questions weight of anyvolumeof liquid
(a) What is the weight of the stone in R.D = weight of equal volumeof water
the air?

104
Student’s Book Form One

Physics Form 1 Final.indd 104 16/10/2024 20:56