Page 219 - Physics

Work, energy and power

At the point of release, the object has When the object reaches the ground, the
potential energy but zero kinetic energy. total energy is given as:
Thus, the total mechanical energy at this
stage is E KE PE   0J 150J 150J  E = KE + PE = 150 J + 0 J = 150 J

When the object is at halfway to the So, while the object was falling its PE
ground (h = 2.5 m). decreased and its KE increased. In this
f
The fi nal PE and KE are: case, its total mechanical energy remained
GPE = mgh constant. The total mechanical energy
f
was therefore conserved.
= 3 kg × 10 N/kg × 2.5 m
= 75 J Conversion of energy can also be
KE = E − mgh studied by considering the motion of a

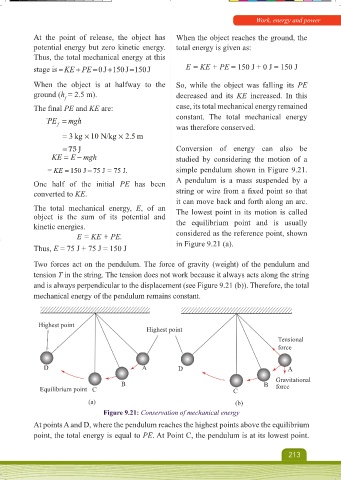
= KE = 150 J − 75 J = 75 J. simple pendulum shown in Figure 9.21.

One half of the initial PE has been A pendulum is a mass suspended by a
converted to KE. string or wire from a fi xed point so that
it can move back and forth along an arc.
The total mechanical energy, E, of an The lowest point in its motion is called
object is the sum of its potential and
kinetic energies. the equilibrium point and is usually
E = KE + PE. considered as the reference point, shown
Thus, E = 75 J + 75 J = 150 J in Figure 9.21 (a).

Two forces act on the pendulum. The force of gravity (weight) of the pendulum and
tension T in the string. The tension does not work because it always acts along the string
and is always perpendicular to the displacement (see Figure 9.21 (b)). Therefore, the total
mechanical energy of the pendulum remains constant.

Highest point (b)
Highest point
Tensional
force

D A D A
Gravitational
B B force
Equilibrium point C C
(a) (b)
Figure 9.21: Conservation of mechanical energy
At points A and D, where the pendulum reaches the highest points above the equilibrium
point, the total energy is equal to PE. At Point C, the pendulum is at its lowest point.

213

Physics Form 1 Final.indd 213 16/10/2024 20:58