Page 100 - Mathematics

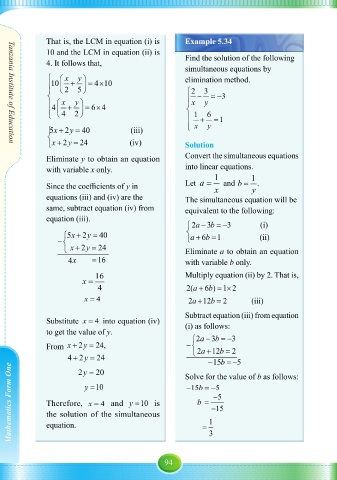
That is, the LCM in equation (i) is Example 5.34
10 and the LCM in equation (ii) is Find the solution of the following
4. It follows that,
simultaneous equations by
  x y  elimination method.
 10  +  = 4 10×
  2 5   2 3
  − = − 3
 4  x + y  = 64  x y
×
   4 2    1 + 6 = 1



5x + 2y = 40 (iii)  x y

 x + 2y = 24 (iv) Solution
Tanzania Institute of Education
Eliminate y to obtain an equation Convert the simultaneous equations
with variable x only. into linear equations.
1
1
Since the coefficients of y in Let a = x and b = y .
equations (iii) and (iv) are the The simultaneous equation will be
same, subtract equation (iv) from equivalent to the following:
equation (iii).
2a −  3b = − 3 (i)
5x +  2y = 40  a + 6b = 1 (ii)
−  
 x + 2y = 24 Eliminate a to obtain an equation
4x = 16 with variable b only.

16 Multiply equation (ii) by 2. That is,
x =
4 2(a + 6 ) 1 2b = ×
x = 4 2a + 12b = 2 (iii)

Subtract equation (iii) from equation
Substitute x = into equation (iv) (i) as follows:
4
to get the value of y. 3b = − 3

From x + 2y = 24, −  2a −  2a + 12b = 2

24
=
4 2y+ 2y = 20 Solve for the value of b as follows:
15b =
−
−
5
Mathematics Form One y = 10 4 and y = 10 is b = 1 − − − 5 5

15b =
−
Therefore, x =
15
the solution of the simultaneous
equation.
=

3
94

25/10/2024 09:51:32
Mathematics form 1.indd 94
Mathematics form 1.indd 94 25/10/2024 09:51:32