Page 103 - Mathematics

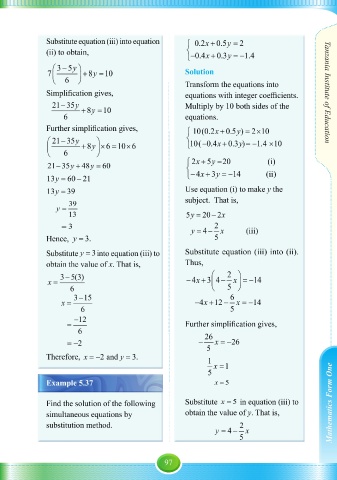
Substitute equation (iii) into equation 0.2x +  0.5y = 2
(ii) to obtain,  1.4
 − 0.4x + 0.3y = −
7   3– 5y   + 8y = 10 Solution
 6  Transform the equations into
Simplification gives, equations with integer coefficients. Tanzania Institute of Education
21 35y− Multiply by 10 both sides of the
+ 8y = 10
6 equations.
Further simplification gives,  10(0.2x + 0.5 ) 2 10y = ×
 21– 35y   10( 0.4x + 0.3 )y = − 1.4 10
−
×
6 10 6

 + 8y ×= × 
 6 
21 35y− + 48y = 60  2x +  5y = 20 (i)
13y = 60 21−  − 4x + 3y = − 14 (ii)
13y = 39 Use equation (i) to make y the

39 subject. That is,
y = 13 5y = 20 2x−

= 3 y = 4 − 2 x (iii)
Hence, y = 3. 5

Substitute y = into equation (iii) to Substitute equation (iii) into (ii).
3
obtain the value of x. That is, Thus,
3 5(3)−  2 
x = − 4x + 3 4−  x =  − 14
6  5 
3 15− 6
x = 6 4x− + 12 − 5 x = − 14

− 12
= Further simplification gives,
6 26

= − 2 − 5 x = − 26
Therefore, x = − 2 and y = 3. 1

5 x = 1
Example 5.37 x = 5

5
Find the solution of the following Substitute x = in equation (iii) to Mathematics Form One
simultaneous equations by obtain the value of y. That is,
substitution method. 2
y = 4 − 5 x

97

25/10/2024 09:51:33
Mathematics form 1.indd 97 25/10/2024 09:51:33
Mathematics form 1.indd 97