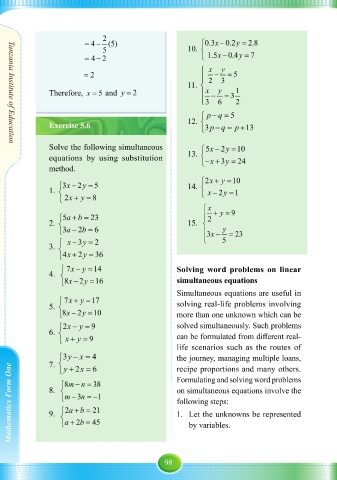
Page 104 - Mathematics

2
4= − (5) 0.3x −  0.2y = 2.8
5 10. 
42= −  1.5x − 0.4y = 7
x
y
= 2  2 − 3 = 5


11. 
2
Therefore, x = and y =  x − y = 3 1
5
  3 6 2
 pq−= 5
Exercise 5.6 12.  3pq−= p + 13

Solve the following simultaneous 5x −  2y = 10
Tanzania Institute of Education
equations by using substitution 13.  −+ 3y = 24
x

method.
y
3x −  2y = 5 14.  2x += 10
1.   x − 2y = 1

y
 2x += 8
23  x += 9
y

2.  5ab+ = 15.  2


 3a − 2b = 6  3x − y = 23
3.  x −  3y = 2   5
 4x + 2y = 36

 7x y−= 14 Solving word problems on linear
4. 
 8x − 2y = 16 simultaneous equations
Simultaneous equations are useful in
 7x y+= 17 solving real-life problems involving
5. 
 8x − 2y = 10 more than one unknown which can be
y
 2x −= 9 solved simultaneously. Such problems
6. 
y
 x += 9 can be formulated from different real-
life scenarios such as the routes of
 3yx−= 4 the journey, managing multiple loans,
7. 
Mathematics Form One 8.  8mn−= 45 1 on simultaneous equations involve the
recipe proportions and many others.
6
2x =
y +

Formulating and solving word problems
38

−
3n =
m −

following steps:
2ab+ =
21

9.
1. Let the unknowns be represented

2b =
a +


by variables.
98
25/10/2024 09:51:34
Mathematics form 1.indd 98 25/10/2024 09:51:34
Mathematics form 1.indd 98